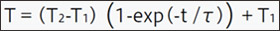Difference between sensor response time and sensor time constant τ (tau) 63.2%.
/Sensor Time Constant (τ)
Responsiveness of any sensor is usually given as a Time Constant and represented by the Greek letter τ “tau”. It is defined as the time required for the sensor reading/output to reach to 63.2% of its total step change in measurand.
EXAMPLE: For a temperature sensor taken out of an ice bath at 0 °C into a room at 10 °C, it will take exactly one time constant (usually given in seconds) to reach 6.32 °C, which is exactly 63.2% of the 10 °C step change in temperature.
Sensor Response Time = 5*τ (5x Time Constant)
The Time Constant of a sensor is very different than its Response Time. In fact, the response time is exactly five times the time constant. Response Time is the time for the sensor reading to reach 99.3% of the total step change in measurand, or in this case the new temperature.
EXAMPLE: For a temperature sensor taken out of an ice bath at 0 °C into a room at 10 °C, it will take exactly five time constants (five times longer) to reach 9.93 °C, which is exactly 99.3% of the 10 °C step change in temperature.
What affects the time constant?
BARANI DESIGN helical solar radiation shield effect on air temperature sensors
Type of media measured. Type of liquid or gas. Since liquids in general have greater heat capacity and higher thermal convection coefficients which reduce the time constant value, they are used in temperature sensor calibrations.
Flow rate of media. In a liquid or gas the time constant is strongly dependent upon the mass flow rate, which must be known when determining the time constant. For temperature sensors in meteorological applications, speed of air flow (wind speed) must defined when defining the time constant for temperature sensors. Solar radiation shields on weather stations significantly reduce the speed of airflow around the sensor, thus changing its practical time constant for each wind speed and radiation shield design.
Emissivity of materials used in sensor construction when compared to its surroundings will change the energy balance of each sensor in each installation. In the case of meteorological air temperature measurement, solar radiation shields are used to limit the amount of error due to the sun’s irradiated heat onto the sensor in the form of global direct radiation, diffuse radiation and reflected radiation. Radiation shield design has a significant effect on the sensor time constant. Different weather conditions will result in different time constants based on the effectiveness of the solar radiation shielding. Please see the calculator of errors in air temperature measurement due to sensor and radiation shield time constants: https://www.baranidesign.com/faq-articles/2019/5/5/the-truth-about-air-temperature-measurement-error-influence-of-sensor-response-time
Thermal conductivity of materials used in sensor construction.
Why a Step Change in Temperature?
A step change is easy to define and easy to measure and independent of experiment time. It is also easy to accurately recreate in a laboratory setting and easy to reproduce consistently and independent of magnitude and is a stable reference point.
Why is τ defined at 63.2%
Simply put, when the elapsed time between any two readings is equal to τ (sensor time constant), the 2nd sensor reading will always be 63.2% closer to the real temperature, no matter at which point in time the sensor values are taken. See Figure 1. The behavior is governed by the following first order equation of a linear time-invariant (LTI) system, where:
T = Current sensor temperature reading/output
T1 = Initial sensor temperature when it was put into new media
T2 = Temperature of the new media that the sensor is measuring
(T2 - T1) = Step change in temperature
t = Elapsed time from the point when the sensor temperature was T1 (usually when the sensor was put into new media)
τ = Sensor time constant (a time value usually in seconds) as given by the manufacturer for the measured media and mass flow rate (will be different for water, air, … and for different media flow rates)
Calculating error due τ 63.2%
To assist in quantifying measurement error due to sensor response time, please see the following web calculator:
Figure 1. How sensor response time relates to the sensor time constant (click to enlarge)
Figure 1 graphically demonstrates how the sensor temperature will approach the “true” temperature of the media asymptotically. Asymptotically means that the sensor reading/output will never truly reach the same temperature as the media. In terms of practical accuracy which is attainable in the real world, the sensor response time is taken as the time taken by the sensor to reach 99.3% of the measured temperature change.
Please note: MeteoHelix IoT Pro micro weather stations have a very low sensor time constant not only due to the design of the helical radiation shield but also due to their optimized sensor tip design.